L’Hospital’s Rule is a useful way to evaluate tricky limits. It is most often used for limits of indeterminate form. The rule is as follows:
If and
are differentiable on some interval around the number
(or if
,
and
are differentiable for all
for some
), and
, then
We can take the derivatives of the numerator and the denominator and then take the limit and it will be the same as the limit of the original ratio. Indeterminate form includes limits of the forms ,
,
,
,
,
and
.
Example 1.
In this example, we see that taking the limit of the numerator and denominator results in , which is an indeterminate form. And since there is not an obvious way to simplify the fraction in order to make the limit easier to compute, it is a good candidate for L’Hospital’s Rule. We take the limit of the numerator and denominator as follows:
Example 2.
Here we see that attempting to take the limit as is results in . So again we use L’Hospital’s Rule:
Taking the limit at this point still results in , so we apply L’Hospital’s Rule again.
is not an indeterminate form and so that is our final answer.
Example 3.
If we plug zero into the limit, we get the indeterminant form . We can’t apply L’Hospital’s Rule right away because we do not have a ratio. Instead, we must manipulate the function so that it becomes a ratio. We can move
to the denominator by rewriting as
.
Now we have a ratio and the indeterminate form . Applying L’Hospital’s Rule,
We still have an indeterminate form , but before applying L’Hospital’s Rule again, we should notice that we can cancel the
in the top and the bottom. It is always best to simplify after applying L’Hospital’s Rule if possible.
If we had tried to use L’Hospital’s Rule before canceling, our ratio would become more complicated with each application of the rule.
Example 4.
Although L’Hospital’s Rule can always be applied to functions that meet the criteria of being differentiable around an interval, it does not always yield a useful answer, even if we see indeterminate forms. Here we will try to use L’Hospital’s Rule.
Noticing that this ratio isn’t any simpler, we can try to apply L’Hospital’s Rule again.
This is the original limit we started with, so we are not getting anywhere using L’Hospital’s Rule. Instead, we should try to simplify our problem by dividing everything by the element with the highest power, in this case .
Noticing that approaches
as
approaches infinity, we can now take the limit
L’Hospital’s Rule is often useful to evaluate limits of indeterminate form. It is most useful when it makes the numerator and denominator simpler than they were before. Remember to always simplify after applying the rule. L’Hospital’s Rule is applicable to all limits of ratios of differentiable functions, but is not guaranteed to make the limit easier to find.
Although the rule was named for Guillaume de l’Hôpital, it was actually discovered by his employee Johann Bernoulli

![Rendered by QuickLaTeX.com \[\lim\limits_{x \to a} \frac{f(x)}{g(x)} = \lim\limits_{x \to a} \frac{f'(x)}{g'(x)}\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-2f323ae6a49c40a960dcaee73330b485_l3.png)
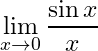
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to 0} \frac{\sin x}{x} = \lim\limits_{x \to 0} \frac{\frac d{dx} (\sin x)}{\frac d{dx} (x)} = \lim\limits_{x \to 0} \frac{\cos x}{1} = \cos(0) = 1\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-1783e93cc7dffaec32aa406b9e773ab8_l3.png)
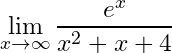
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to \infty} \frac{e^x}{x^2+x+4} = \lim\limits_{x \to \infty} \frac{\frac d{dx} (e^x)}{\frac d{dx} (x^2+x+4)} = \lim\limits_{x \to \infty} \frac{e^x}{2x+1}\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-aed2d8cc5d2227bf160218341194efd3_l3.png)
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to \infty} \frac{e^x}{2x+1} = \lim\limits_{x \to \infty} \frac{\frac d{dx} (e^x)}{\frac d{dx} (2x+1)} = \lim\limits_{x \to \infty} \frac{e^x}{2} = \infty\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-a4ecbbacf70fd2add83b5da8571cb5a8_l3.png)
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to 0} (x \cdot \ln x) = \lim\limits_{x \to 0} \frac{\ln x}{\frac {1}{x}}\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-f726e0bec597bcfb76dfd2a8d7295abe_l3.png)
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to 0} \frac{\ln x}{\frac {1}{x}} = \lim\limits_{x \to 0} \frac{\frac d{dx} (\ln x)}{\frac d{dx} (\frac {1}{x})} = \lim\limits_{x \to 0} \frac{ \frac {1}{x}}{- \frac {1}{x^2}}\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-51c8a71b0459f0189a5b8297854141cd_l3.png)
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to 0} \frac{ \frac {1}{{\cancel {x}}}}{- \frac {1}{x^{\cancel{2}}}} = \lim\limits_{x \to 0} \frac {1}{- \frac{1}{x}} = \lim\limits_{x \to 0} (-x) = 0\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-bdd92ff75172615778ee5fd3a96e99fe_l3.png)
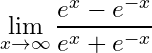
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to \infty} \frac {e^x - e^{-x}}{e^x + e^{-x}} = \lim\limits_{x \to \infty} \frac {\frac d{dx} (e^x - e^{-x})}{\frac d{dx}(e^x + e^{-x})} = \lim\limits_{x \to \infty} \frac {e^x + e^{-x}}{e^x - e^{-x}}\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-80fd32b55f6f7f962533ad952948b58c_l3.png)
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to \infty} \frac {e^x + e^{-x}}{e^x - e^{-x}} = \lim\limits_{x \to \infty} \frac {\frac d{dx} (e^x + e^{-x})}{\frac d{dx}(e^x - e^{-x})} = \lim\limits_{x \to \infty} \frac {e^x - e^{-x}}{e^x + e^{-x}}\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-7f19f727b3ce804ce046428ec9d8407b_l3.png)
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to \infty} \frac {e^x - e^{-x}}{e^x + e^{-x}} = \lim\limits_{x \to \infty} \frac {\frac {e^x}{e^x} - \frac {e^{-x}}{e^x}}{\frac {e^x}{e^x} + \frac{e^{-x}}{e^x}} = \lim\limits_{x \to \infty} \frac {1 - e^{-2x}}{1 + e^{-2x}}\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-837133267670d36bbb12f7d69fbf005c_l3.png)
![Rendered by QuickLaTeX.com \[\lim\limits_{x \to \infty} \frac {1 - e^{-2x}}{1 + e^{-2x}} = \frac {1-0}{1+0} = 1\]](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-f89a35443fc1ffcc4189894ee7495040_l3.png)