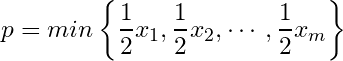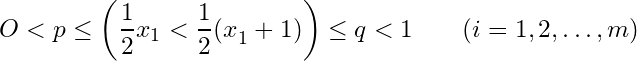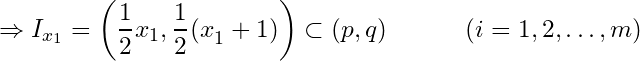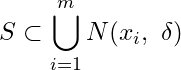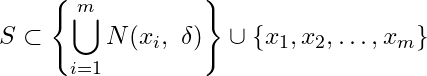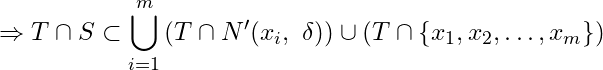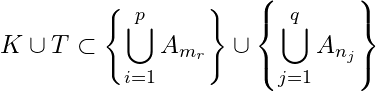INTRODUTION
In advance analysis, the notion of ‘Compact set’ is of paramount importance. In , Heine-Borel theorem provides a very simple characterization of compact sets. The definition and techniques used in connection with compactness of sets in
are extremely important. In fact, the real line sets the platform to initiate the idea of compactness for the first time and the notion of compactness plays its important role in topological spaces.
The definition of compactness of sets in uses the notation of open cover of sets in
. For this propose we need some definitions and illustrative examples to clear the meaning of cover of a set in
.
COVER, OPEN COVER, SUB COVER
Definition (Cover): Let be subset of
and
be a collection of sub sets of
.
is said to cover
or, in other words,
is said to be a covering of
if
i.e. if for some
.
If , for each
, is an open set and

is said to be an open cover of
.
For example
- (i) The family
is an open cover of
, since
and the sets in family
are open sets.
- (ii) The family
is a collection of open sets in
, but
is not a cover of
, since
and
do not belong to
.
- (iii) The family
is a collection of open sets in
and
for some
. Hence
is an open cover of
.
Note: If be a collection of open intervals
in
such that
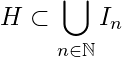
is also an open cover of
.
Definition (Sub-Cover): Let and
be a collection of sets in
which covers
. If
be a sub-collection of such that
itself is a cover of
then
is said to be a sub cover of
. If
is a finite sub collection of
such that
is a cover of
then
is said to be a finite sub cover of
.
For example if then
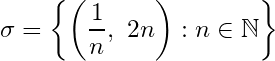
.
If 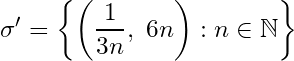
and
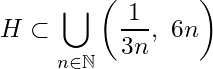
is an open sub cover of
.
Definition (Countable set): set
in
is said to be a countable set if either it is finite or if it is infinite, it is enumerable i.e. there exists a bijective mapping from
to
.
For example
- (i) every finite set is countable,
- (ii)
are all countable sets,
- (iii) the sets
are not countable sets.
Definition (Countable Sub cover): Let and
be a collection of sets in
such that
covers
. If
be a countable sub-collection of
such that
covers
then
is said to be a countable sub cover of
.
For example, if then
is an open cover of
and
is a countable sub-collection of
, since if
, the set
has one-to-one correspondence with
.
also covers
. Hence
is a countable sub cover of
. Note that there are infinitely many countable sub covers of
, since
is a countable set and
is the set of open intervals with rational end points and hence
itself is a countable family of open sets so that every sub cover of
is countable.
We now give some examples of open cover of a set in which has no finite sub cover.
Example 1. Let and
where
. Show that
is an open cover of
but it has no finite sub cover.
Solution: Let . Then
and by the Archimedean property of
, there exists a natural number
such that
for some
. Hence
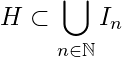
is a collection of open sets in
which covers
i.e
is an open cover of
.
If possible, let where
are natural numbers such that
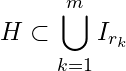
covers
.
Let then
and for
Since but
, so we have a contradiction. Hence
is not a cover of
.
Thus there exists no finite subcollection of that will cover
.
Example 2. Let and
. Show that
is an open cover of
, but it has no finite sub cover.
Solution: Let . Then
. By the Archimedean property of
there exists a natural number
such that
for some

is an open cover of
(
is also a countable cover of
).
If possible, let where
are natural numbers such that
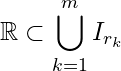
is a sub cover of
.
Let . Then
and
for all
.
Thus 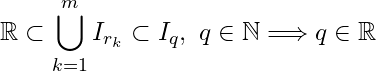
, a contradiction.
Thus it is proved that no finite subcollection of can cover
.
Example 3. Let and

. Show that
is an open cover of
but no finite subcollection of
can cover
.
Solution: Let . Then
. For

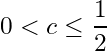
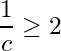
, there exists a natural number
such that
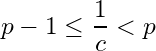
,

for some natural number
.
Hence 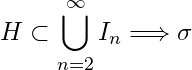
.
If possible, let be a finite subcollection of
, where
are natural numbers
, such that
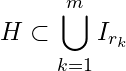
Let and
then
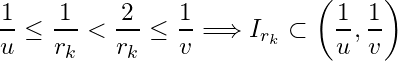
.
Thus 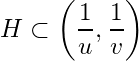
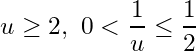
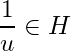
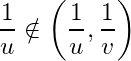
covers
.
Example 4. Let and
. Let
where
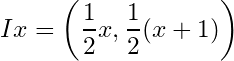
is an cover of
but it has no finite sub cover.
Solution: Let . Then
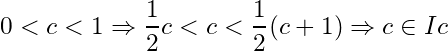
Hence 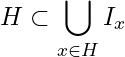
is an open cover of
.
If possible, let where
for
and
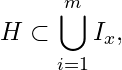
Let
And 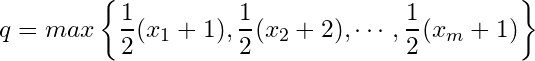
Then
. Since
but they do not belong to
, we have a contradiction. Hence
has no finite sub-collection that can cover
, i.e.
has no finite sub cover.
Example 5. The collection of open intervals is an uncountable cover of
but
where
is the set of integers, is countable sub cover of
.
Solution: Let . Since
is both unbounded above and unbounded below, thee always exist two real numbers
and
such that
such that
in
such that
. Hence
.
Thus . As open interval
is an uncountable subset of
, so
is an uncountable cover of
.
Thus . Then by the Archimendeon property of real numbers, there exists an integer
such that
. This implies
for some
. Hence
. Thus
is a countable sub cover of
.
COMPACT SETS IN 
Definition (Compact set): set
(
) is said to be a compact set in
if every open cover of
has a finite sub cover. More explicitly,
is said to be compact if for any open cover
of
, there is a finite sub collection
of
such that
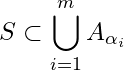
is a finite sub-cover of
.
NOTE: To prove that a set is compact in
, we must examine an arbitrary collection of open sets whose union contains
, and show that
is contained in the union of some finite number of sets in the given collection, i.e. we must have to show that any open cover of
has a finite sub-cover. But to prove that a set
is not compact, it is sufficient to choose one particular open cover
has no finite sub-cover, i.e. union of any finite number of sets in
fails to contain
.
Theorem 1 (Heine-Borel Theorem) :
Statement:– close and bounded subset of
is a compact set in
, or in other words every open cover of a closed and bounded subset of
has a finite sub cover.
Proof, Let be a closed and bounded subset of
.
Let be an open cover of
. We assume that
has no finite sub-cover. Then
is not a subject of the union of finite number of open sets in
.
Since H is a bounded subject of , there exist real number
such that
.
Let . If
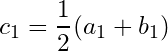
and
are subset of the union of finite number of open sets in
, for otherwise both
and
are subsets of the union of finite number of open sets in
contains
, contradicting our assumption that
has no finite sub-cover.
We call or
according as
and it is not a subset of the union of finite number of open sets in
or
and it is not a subset of the union of finite number of open sets in
.
Let and
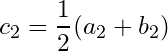
and
is non-empty and it is not a subset of the union of finite number of open sets in
. If the first subset is non-empty and it is not a subset of the union of finite number of open sets in
, we call
, otherwise we call
.
Let , and
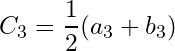
Continuing this process of bisection of intervals, we have a family of close and bounded intervals such that
, for all
,
For all is non-empty and it is not a subset of the union of finite number of open sets in
.
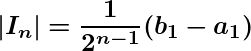
as
.
Then by Nested Interval Theorem, 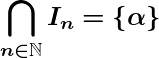
.
Since , for any positive
, there exists a natural number
such that
i.e.
and
. Hence
. Since
and it is not a subset of the union of finite number of open sets in
,
contains infinite number of elements of
is a limit point of
. Since
is closed,
.
Now for some
.
is an open set, hence there exists a positive
and hence
. Since
is an open cover of
,
for some
, which goes against the construction of (
.
Hence our assumption that is not a subset of the union of finite number of sets in
is wrong and it is established that if
is closed and bounded, any open cover
of
has a finite sub cover so that
is a compact set in
.
Remark: In the Heine-Borel theorem neither of the two conditions (i) is closed (ii)
is bounded can be dropped. The theorem fails if one of the two conditions is withdrawn – this is evident if we go through the example 1.2.3 and the example 1.2.1. In example 1.2.3,
is closed but bounded and in example 1.2.1,
is closed but no bounded.
Thus both the conditions (i) and (ii) are necessary for a set in to be compact. Next we shall show that these two conditions are also sufficient for a set
to be compact in
.
Theorem 2 (Converse of Heine-Borel Theorem):
Statement:– compact subset of
is closed and bounded in
.
Proof. Let be a compact in
. First we shall prove that
is a closed set in
.
Let and
. Then exist two positive numbers
and
such that
.
Let .
Then is an open cover of
is compact,
has a finite sub cover i.e. there exist elements
of
and positive numbers
such that
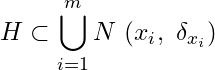
there exists positive numbers
such that
.
Let .
Then . Therefore
is an interior point of
.
Since is arbitrary point of
,
is open. Hence
is closed.
Nest we shall prove that is bounded.
Let be a fixed positive number. Then
is an open cover of
. Since
is compact,
has a finite sub-cover. Then there exist points
of
such that
is a finite cover of
. If
and
then
![Rendered by QuickLaTeX.com H \subset \bigcup_{i=1}^{m}N(x_i, \delta) \subset [p - \delta, q + \delta] \Rightarrow H](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-99fe79b62f44214eed641b5b3370f6d7_l3.png)
Hence it is proved that if is a compact set in
, it is closed and bounded in
. This completes the proof.
Combining the theorems 1 and 2 we have the following theorem which gives a complete characterization of compact sets in .
Note: – set in
is compact if and only if is closed and bounded in
.
Definition (Heine-Borel Property): set
(
) is said to possess Heine-Borel property if every open cover of
has a finite sub cover.
set is said to be compacted if it has the Heine-Borel property.
Example 6. Using the definition of compact set, prove that the set is not compact although it is a closed set in
.
Solution: In example 1.2.1, it is shown that , where
, is an open cover of
and
has no finite sub cover. Hence from definition
is not compact.
is a closed set in
, since
is open.
Note: In the example 1.3.1, does not satisfy Heine-Borel property, since
is not bounded in
.
Example 7. Using definition of compact set show that a finite subset of is a compact set in
.
Solution: Let be a finite subset of
. Let
be an open cover of
. Then each
is contained in some open set
of
for some
. Let
. Then
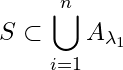
also covers
.
Hence is a finite sub cover of
. Therefore, by definition,
is a compact set in
.
Theorem 3.
Statement:– If be a compact subset of
, then every infinite subset of
has a limit point belonging to
.
Let be an infinite subset of the compact subset of
of
such that
has no limit point belonging to
.
Let . Then
is not a limit point of
. There exists a positive
such that
where
, called deleted
.
Let , which is a collection of open sets in
. Since

is an open cover of
.
Since is compact, there exists a finite sub collection
of
where
and
such that
covers
i.e.
i.e.
[Since and
for
]
which shows that is a finite subset of a compact set
in
has a limit point in
.
Theorem 4.
Statement: – If be such that every infinite subset of
has a limit point in
then
is closed and bounded in
.
Proof. First we shall prove that is bounded. If possible, let
be unbounded above. Let
be any point of
. Since
is unbounded above, there exists a point
in
such that
. By similar argument there exists a point
in
such that
and so on. Continuing this process indefinitely we ultimately have an infinite subject
of
, which being a discrete set, has no limit point in
is a bounded above subset of
. Similarly, If
is unbounded below we can construct an infinite subset of
which has no limit point. Hence
is also bounded below so that
is a bounded subset of
.
Next we shall prove that is closed in
.
Since S is an infinite and bounded subset of , by the Bolzano-Weierstrass theorem on set,
has a limit point in
.
Let be a limit point of
. Then for any
is infinite.
For is infinite. Let us take a point
.
For 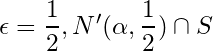
,
such that 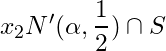
of
such that
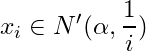
. We shall show that
has a unique limit point which is
.
Let be any positive number. Then by the Archimedean property of
, there exists a natural number
such that
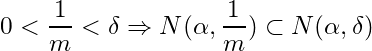
contains infinite subset
of
. Thus for every positive
,
is infinite which proves that
is a limit point of
.
To prove uniqueness, let be a limit point of
. Let
. Then the neighborhoods
and
are disjoint (since either
or
). By the Archimedean property of
, there exists a natural number
such that
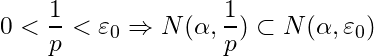
belongs to
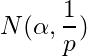
contains all elements of
expect some finite number of elements and hence
can contain almost finite number of elements of
. This implies
is not a limit point of
. Hence
is the only limit point of
. By the condition of the theorem
. Hence
is closed.
Thus it is proved that is closed and bounded in
.
Note: – subset
of a compact subset of
is compact if and only if every infinite subset of
has a limit point belonging to
.
Theorem 5.
Statement: – A Subset of
is compact if and only if every sequence in
has a subsequence that converges to a point in
.
Proof. Let be compact. Then
is closed and bounded.
Let be a sequence of points in
. Since
is bounded,
is bounded. By the Bolzano-Weierstrass theorem on sequence, there exists a subsequence
of
that converges to a point, say
. Since
is closed, if
,
and
is open. Then there exists a neighborhood
of
which contains no point of
. This implies
contains no element of the sequence
which contradicts that
. Thus
. Hence every sequence in
has a subsequence converging to a point of
.
Suppose is not closed. Then
has a limit point, say
which is not in
. Since
is a limit point of
, there is a sequence
in
, where
for all
, such that
. Then every subsequence of
converges to
. Since
, there is no subsequence of
that converges to a point of
.
Suppose is not bounded. Then there exists a sequence
in
such that
for all
. Then every subsequence of unbounded sequence
is unbounded and hence no subsequence of
converges to a point in
.
Hence, by contrapositive argument, it is proved that if every sequence in has a subsequence that converges to a point of
the
is closed and bounded and hence by Heine-Borel theorem
is compact.
Note: Following theorem 5., an alternative definition of compact set can be given in the from:
“A set in
is called a compact set in
if every sequence in
has a subsequence that converges to a point of
.”
Theorem 5 and the Heine-Borel theorem together prove the equivalence of the two definitions.
Example 8. If is a closed subset of a compact set
in
then using definition of compact set, prove that
is compact.
Solution: is open, since
is closed.
Let be an open cover of
. Suppose
is not an open cover of
. Let
. Then
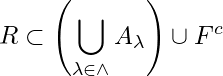
is an open cover of
. Since
,
is also an open cover of
.
being compact,
has a finite sub collection
such that
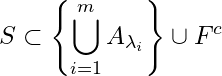
.
must contain
, otherwise
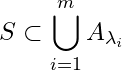
is an open cover of
, which is contrary to our assumption.
Since we have
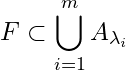
Which shows that,
is a finite sub collection of
and
covers
, which implies
is a finite sub cover of
. Therefore, by definition,
is compact.
Example 9. Every compact set in has greatest as well as least element.
Solution: Let be any compact set in
. If possible, let
have no greatest element. Then for each element
. let
. Then
is an open set. Let
, a family of open sets in
. Let
. Since
has no greatest element, there exists an element
in
such that
. Thus
. Hence
is an open cover of
.
being compact,
has a finite sub-cover, say
.
Let Then
for
and
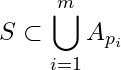
.
Then and
. This leads to a contradiction, since
. Hence our assumption is not tenable and
has greatest element.
To prove the next part, let, if possible, has no least element. Then for each element
, let
is an open set. Let
, a family of open sets in
. Let
. Since
has no least element there exists an element
in
such that
. Then
and
. Thus
is an open cover of
. Since
is compact,
has a finite sub collection
that covers
.
Let where
and
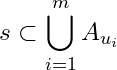
then
.
Now but
which contradicts our assumption. Hence
has least element.
Example 10. If and
are component sets in
, show that
is also compact. Give an example to show that union of an infinite number of compact sets in
is not necessarily a compact set in
.
Solution: Let be a family of open sets in
such that
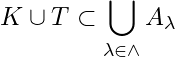
is an open cover of
.
Since 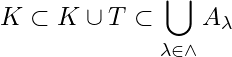
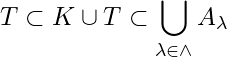
is an open cover of both
and
. Since
and
are both compact sets in
, then there exist two finite sub collections
and
of
such that
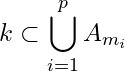
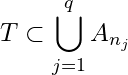
and
.
Let . Then
is a finite sub collection of
such that
or
for some
Or for some
Hence
cover
. Hence, from definition,
is compact.
Second Part.
Let . Then for each
,
is closed and bounded set in
and by Heine-Borel Theorem,
is compact for every
. Thus
is an infinite collection of compact sets in
.
But 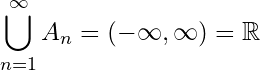
is not necessarily compact.
Example 11. Let be a closed subset of
and
be a component subset of
. Prove that
is component.
Solution: Since is compact, by converse of Heine-Borel theorem
is closed.
being closed,
is a closed subset of compact set
. Then following exactly similar arguments given in example 1.3.3 (replacing
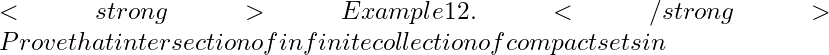
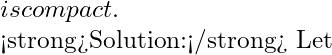
\mathbb{R}
S =\ \bigcap_{C\in\sigma} C
C
\sigma
S
R
\mathbb{R}-S
g’ = \{G : \alpha \in \Lambda\}
\mathbb{R}
S
S \subset \ \bigcap_{\alpha\in\land} G_\alpha
g
C
C \in \sigma
g’
\{G : \alpha \in \Lambda\}
\mathbb{R} – S
\mathbb{R} \subset \left\lbrace\bigcup_{\alpha\in\land} G_\alpha\right\rbrace \cup \left(\mathbb{R} – S\right)
C \subset \mathbb{R}
C \subset \left\lbrace \bigcup_{\alpha\in\land} G_\alpha\right\rbrace \cup \left(\mathbb{R} – S\right)
C \in \sigma
g’
C
C \in \sigma
C
C \in \sigma
g”
g’
g”
C
C \in \sigma
f” = \{G_{\alpha_1},\ G_{\alpha_2}, \ldots,\ G_{\alpha_m},\ \mathbb{R}\ – S\}
g”
\mathbb{R} – S
C \subset \bigcup_{i=1}^{m}G_{\alpha_i}
g
C
C \in \sigma
C \subset \left\lbrace\bigcup_{i=1}^{m}G_{\alpha_i}\right\rbrace \cup (\mathbb{R} – S)
C \in \sigma
S \subset C
S \subset \bigcup_{i=1}^{m}G_{\alpha_i}
g”’ = \{G_{\alpha_1},\ G_{\alpha_2}, \ldots,\ G_{\alpha_m}\}
g”’
g
S
g
S
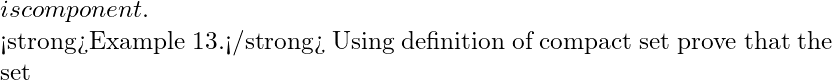
\mathbb{R}
I_n = \left(\frac{1}{n+1},\ \frac{n+1}{n}\right), n \in \mathbb{N}
\sigma = \{I_n : n \in \mathbb{N}\}
\sigma
\mathbb{R}
x \in (0, 1]
x = 1
x \in I_n
n \in \mathbb{N}
0< x < 1
\mathbb{R}
m
m \le\ \frac{1}{x} < m + 1
\Rightarrow \frac{1}{m+1} < x \le\ \frac{1}{m} <\ \frac{m+1}{m} \Rightarrow x \in I_mm \in \mathbb{N}
(0, 1] \subset \bigcup_{n\in\mathbb{N}} I_n
\sigma
(0, 1]
\sigma’ = \{I_{r_1},\ I_{r_2}, \ldots,\ I_{r_m}\}
\sigma
r_1, r_2, \ldots, r_m
(0, 1] \subset \bigcup_{k=1}^{m}I_{r_k}
u = \max \{r_1 + 1, r_2 + 1,\ldots, r_m + 1\} \Rightarrow u \geq r_k + 1
k = 1, 2, \ldots, m
v = \min \{\frac{r_1}{r_1+ 1},\ \frac{r_2}{r_2+ 1}, \ldots,\ \frac{r_m}{r_m+\ 1}\} \Rightarrow v \leq \frac{r_k}{r_k+ 1}
k = 1, 2, \ldots, m
0 < \frac{1}{u} \leq \frac{1}{r_k+ 1} < \frac{r_k+ 1}{r_k} \leq \frac{1}{v},\ x = 1, 2,\ldots, m\Rightarrow I_{r_k} \subset \left(\frac{1}{u},\ \frac{1}{v}\right)
k = 1, 2,\ldots, m\Rightarrow (0, 1] \subset \left(\frac{1}{u},\ \frac{1}{v}\right)
0 < \frac{1}{u} < 1
\frac{1}{u} \in (0, 1]
\frac{1}{u} \left(\frac{1}{u},\ \frac{1}{v}\right)
\sigma
(0, 1]
(0, 1]
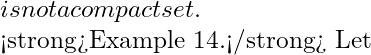
\Gamma = \{(x – \varepsilon, x + \varepsilon), \varepsilon > 0\}
\Gamma
[a, b]
a < x < b
\Gamma = \{I_x : a < x < b\}
x
(a, b)
I_x
\mathbb{R}
x_1, x_2 \in (a, b)
a < x_1 < a + \varepsilon
b – \varepsilon < x_2 < b
x_1 – \varepsilon < a,\ x_1 + \varepsilon > a
b < x_2 + \varepsilon, x_2 – \varepsilon < b
a \in I_{x_1}
b \in I_{x_2}
[a, b] \subset \bigcap_{a<x<b} I_x \Rightarrow \Gamma
[a, b]
[a, b]
\Gamma
S
A_\alpha
\mathbb{R}
\Lambda
S
S \subset \bigcup_{\alpha\in\land} A_\alpha
x \in S
\alpha(x) \in \Lambda
x \in A_{\alpha(x)}
A_{\alpha(x)}
x
I(x)
x
x \in I(x) \subset A_{\alpha(x)}
I(x)
J(x) \subset I(x)
x \in J(x)
j(x)
S \subset \bigcup_{x\in S} I\left(x\right)
\{J(x) : x \in S\}
S
\{J(x) : x \in S\}
C’ = \{j_1, j_2, j_3, \ldots\}
S
J_n \in C’
x_n \in S
x_n \in J_n \subset I(x_n) \subset A_{\alpha_n}
J_n \in C’
A_{\alpha_n} \in C
C” = \{A_{\alpha_1},\ A_{\alpha_2}, \ldots,\ A_{\alpha_n}, \ldots\}
C”
C
S

T
S
T = \left\lbrace 0,\ \frac{1}{m}\ :m\ \in\ N_1\right\rbrace
T = \left\lbrace\frac{1}{m}\ :m\ \in\ N_1\right\rbrace
N_1
\mathbb{N}
0
T
0 \in S
S
S
S
S
\mathbb{R}
A = \left\lbrace\frac{1}{m}+\frac{1}{n}\ :m\ \in\ \mathbb{N},\ n\ \in\mathbb{N}\right\rbrace
B = \left\lbrace 0,\ \frac{1}{m}\ :m\ \in\ \mathbb{N}\right\rbrace
C = \{0\}
S = A \cup B \cup C
\mathbb{R}
S \subset [0, 2]
A,\ B,\ C
A’ =\left\lbrace 0,\ \frac{1}{m}\ :m\ \in\ \mathbb{N}\right\rbrace
B’ = \{0\}
C’ = \emptyset
S’ = A’ \cup B’ \cup C’ = \{0\} \cup \left\lbrace\frac{1}{m}\ :m\ \in\ \mathbb{N}\right\rbrace = B \cup C \subset S
S
S
\mathbb{R}
S
\mathbb{R}
f : \mathbb{N} \to B
f(m) = \frac{1}{m},\ m \in\ \mathbb{N}
B
S’
S’
\mathbb{R}

\mathbb{R}
\bigcap_{n\in\mathbb{N}} F_n
F_n \supset F_{n+1}
n \in \mathbb{N}
F_n
n \in \mathbb{N}
F_n \neq \emptyset
n \in \mathbb{N}
x_n \in F_n
\{x_n\}_n
x_n \in F_n \subset F_m
m \leq n
X
\{x_n\}_n
X
\alpha \in X
x_n = \alpha
n
\mathbb{N}
p \in \mathbb{N}
k \geq p
x_k = \alpha
\alpha = x_k \in F_k \subset F_p
p \in \mathbb{N}
\alpha \in \bigcap_{n\in\mathbb{N}} F_n \Rightarrow \bigcap_{n\in\mathbb{N}} F_n \neq \emptyset
X
F_n
F_1 \supset X
X
X
\beta
m
\beta
X
\beta
N(\beta)
X
N(\beta)
x_n
x_n \in F_m
n \geq m
N(\beta) \cap F_m
\beta
F_m
F_m
\beta \in F_m
m \in \mathbb{N}
\beta \in \bigcap_{n\in\mathbb{N}} F_n \Rightarrow \bigcap_{n\in\mathbb{N}}{F^\prime}_n \neq \emptyset
\bigcap_{n\in\mathbb{N}}{F^\prime}_n
E
\mathbb{Q}
E
\mathbb{Q}
x_1
y_1
E
\subset \mathbb{Q}
\sqrt{2} < x_1 < y_1 < \sqrt{3}
x_2
y_2
E
\sqrt{2} < x_2 < x_1 < y_1 < x_2 < \sqrt3
E
\{x_n\}_n
\{y_n\}_n
\{x_n\}_n
\sqrt{2}
\{y_n\}_n
\sqrt3
\sigma = \{I_n : n \in \mathbb{N}\}
I_n = (x_n,\ y_n)
\sqrt2 < x_n < y_n < \sqrt3
n \in \mathbb{N}
x_n \in \mathbb{Q}
y_n \in \mathbb{Q}
m \in \mathbb{N}
x \in E
x \in I_m
m \in \mathbb{N}
E \subset\bigcup_{n\in\mathbb{N}} I_n
\sigma
E
\sigma’ = \{I_{r_1},\ I_{r_2}, \ldots,\ I_{r_m}\}
\sigma
\sigma’
E
r_1, r_2,\ldots, r_m
E \subset \bigcup_{i=1}^{m}I_{r_i}
p = \max \{r_1, r_2,\ldots, r_m\}
p \in \mathbb{N}
I_{r_i} \subset I_p
I = 1, 2, \ldots, m
E \subset I_p = (x_p,\ y_p)
\sqrt2 < y_p < \sqrt3 \Rightarrow y_p \in E
y_p \notin I_p
\sigma
E
\mathbb{Q}
E
\mathbb{Q}
x \in E \Rightarrow 1 < \sqrt2 < x < \sqrt3 < 2
1
2
E
\mathbb{Q}

\mathbb{Q}
p < \sqrt2
\mathbb{R}
r
p < r < \sqrt2
\delta
p
\delta = r – p > 0
(p – \delta, p + \delta)
E
p + \delta = r < \sqrt2
(p – \delta, p + \delta) \cap E = \emptyset \Rightarrow p
E
q > \sqrt3
\mathbb{R}
\sqrt3 <s < q
\delta’ = q – s > 0
\delta’
q
(p – \delta’, p + \delta’)
E
q – \delta’ = s > \sqrt3
(p – \delta’, p + \delta’) \cap E = \emptyset \Rightarrow q
E
E
E
E
E
E
\mathbb{Q}
\mathbb{Q}
\mathbb{R}
\sigma
\mathbb{R}
E
\sigma
\sigma’
\sigma’
\sigma’
\sigma’ = \{I_n : n \in \mathbb{N}\}
\sigma’
E
E \subset\bigcup_{n\in\mathbb{N}} I_n
A_n = E \cap \left(\bigcup_{k=1}^{n}I_k\right)^c = E – \bigcup_{k=1}^{n}I_k (n = 1, 2, 3,\ldots.)
A_n \supset A_{n+1}
n \in \mathbb{N}
\bigcup_{k=1}^{n}I_k
E
\mathbb{R}
n \in \mathbb{N}
A_n
\mathbb{R}
\{A_n\}

n \in \mathbb{N}
\bigcap_{n\in\mathbb{N}} A_n \neq \emptyset \Rightarrow
c \in \bigcap_{n\in\mathbb{N}} A_n \Rightarrow c \in E
c \notin I_n
n \in \mathbb{N}
c \notin \bigcup_{n\in\mathbb{N}} I_n
\sigma’
E
A_m = \emptyset \Rightarrow E \subset \bigcup_{k=1}^{m}I_k
\sigma” = \{I_1, I_2, \ldots, I_m\}
\sigma’
\sigma
E
E
E
S \subset \mathbb{R}
S
c \in S
f
c
\varepsilon> 0
(\varepsilon, 0) > 0
f(c)\ – \varepsilon < f(x) < f(c) + \varepsilon
c – \delta < x < c + \delta
x \in S
f(x)\ \in\ N(f(c),\ \varepsilon)
x \in N(c, \delta) \cap S
f
S
S
S \subset \mathbb{R}
f : S \to \mathbb{R}
S
f
S
S
\mathbb{R}
f(S)
\mathbb{R}
f\left(S\right)
f(S)\ \subset\ \bigcup_{\alpha\in\Lambda} I_\alpha
\sigma
f(S)
a \in S
f(a)\ \in\ f(S)
I_{\alpha’}
\sigma
f(S) \in I_{\alpha’}
I_{\alpha’}
f(a)
\varepsilon_a
\delta_a
f(x)\ \in\ N(f(a),\ \varepsilon_a)\ \subset\ I_\alpha’
x \in N(a,\delta_a) \cap S
C = \{N(a, \delta_a) : a \in S\}
S
S
C
C’ = \{N(a, \delta_a) : i = 1, 2, \ldots, m\}
a_i \in S
\delta_{a_i} > 0
i = 1, 2, \ldots, m
C’
S
S \subset \bigcup_{i=1}^{m}{N(a_i,\ \delta_{a_i})}
f(S)
f(x)
x \in S
x \in N(a_p,\ \delta_{a_p})
p = 1, 2, \ldots, m
x \in N(a_p, \varepsilon_{a_p}) \cap S
p = 1, 2, \ldots, m
f(x) \in\ N(f(a_p),\ \varepsilon_{a_p})\ \subset\ I_{{\alpha’}_p}
p = 1, 2, \ldots, m
f(x) \in\ f(S)\ \Rightarrow\ f(x) \in\ I_{{\alpha’}_p}
p = 1, 2, \ldots, m
f(S) \subset\ \bigcup_{P=1}^{m}{\ I_{{\alpha’}_p}}
\sigma’ = \{{I}_{{\alpha’}_1},\ I_{{\alpha’}_2}, \ldots,\ I_{{\alpha’}_m}\}
\sigma
f(S)
\sigma’
\sigma
f(S)
f(S)
\mathbb{R}
\mathbb{R}
\sup{f(S)}
f(S)
f(S)

\mathbb{R}
f : S \to \mathbb{R}
S (\subset R)
f(S)
S
\mathbb{R}
S = \left\lbrace x \in (0, 1): x \neq \frac{1}{n}, n \in \mathbb{N}\right\rbrace
\sigma = \left\lbrace \left(\frac{1}{n+1},\ \frac{1}{n}\right) : n \in \mathbb{N}\right\rbrace
\sigma
S
\sigma
S
A = \left[\frac{1}{2},\ \frac{7}{2}\right]
B =\left(1,\ \frac{9}{2}\right)
A \cup B
A
\mathbb{R}
B
\mathbb{R}
A \cup B
\mathbb{Q}
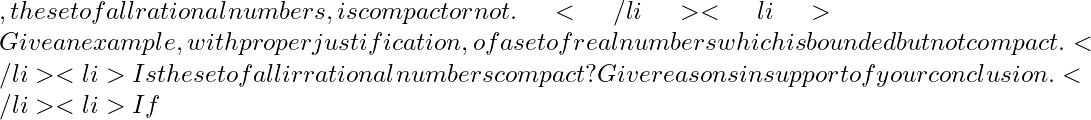
B
\mathbb{R}
A \cap B
\mathbb{R}
\mathbb{R}$?
Bibliography
- An introduction to functional analysis, By Charles Swartz.
- Introduction to Topology, By Bert Mendelson.
- The Elements of Real Analysis, R.G
- Real Analysis, H.L. Royden
Thank you for reading this article. We will meet next time with a new article
Also, you may want to check our blog posts about Fourier Series, Riemann Integral, Lebesgue Outer Measure, and All The Logarithm Rules You Know and Don’t Know About
And don’t forget to join us on our Facebook page stay updated on any new articles and a lot more!!!!!

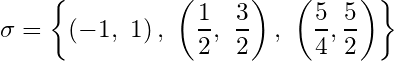 is an open cover of
is an open cover of ![Rendered by QuickLaTeX.com \left[0,\ 2\right]\subset\left(-1,1\ \right)\cup\left(\frac{1}{2},\frac{3}{2}\right)\cup\left(\frac{5}{4},\frac{5}{2}\right)](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-19570859c75ffdb815edcb35b7dfeddf_l3.png) and the sets in family
and the sets in family 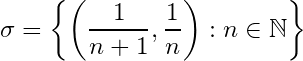 is a collection of open sets in
is a collection of open sets in 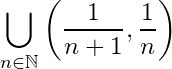 .
.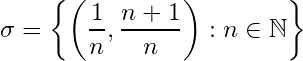 is a collection of open sets in
is a collection of open sets in ![Rendered by QuickLaTeX.com x\in(0,\ 1]\Rightarrow x\in \left(\dfrac{1}{m},\dfrac{m+1}{m}\right)](https://www.mathacademytutoring.com/wp-content/ql-cache/quicklatex.com-dff6d44c5792dacea34c1aef76e75633_l3.png) for some
for some