If you took a look at the previous articles about limits of functions and their properties, we used many times the notion of infinity and evaluated the limits when approaching infinity. In this article, we will learn about the concept of infinity and some of its characteristics, counterintuitive facts, paradoxes, and more!!!

Introduction:

Infinity, a word that we all hear and may think we comprehend, but do we?! Or do we just have the impression or even the illusion of understanding it?! Well, one thing to start with is that the abstract concept of infinity took a long time and effort to form even from the greatest minds of mathematicians and philosophers to arrive at the present understanding, so chances are we have a lot of things to learn about infinity.

Infinity is an abstract concept describing what is uncountable, unlimited, endless, or without boundaries, the word infinity comes from the Latin word “infinitas”, which means “boundless”. Infinity is usually noted with the symbol called “Lemniscate” originating from the Latin word “lemniscus” which translates to “ribbon”, this symbol was introduced for the first time by the English mathematician John Wallis around 1655.

Although a theoretical concept, it is of the utmost importance in many fields like mathematics, physics, computing, or even in metaphysical or religious subjects.

Humans started thinking about infinity since the Greeks and ancient Indians or maybe even before. The idea of infinity took a long time to develop to the current understanding, many different philosophers, mathematicians, and physicists contributed throughout the centuries each from their perspective and fields of knowledge.

In mathematics the concept of infinity wasn’t always welcome through time, some mathematicians avoided the idea of infinity, and some wanted to banish it from mathematics to avoid the puzzling questions and the counterintuitive ideas and cases that come along with it, and to avoid the trouble of fitting the infinity in the world of mathematics. But with the work and persistence of some mathematicians to formalize it, and after recognizing that infinity has its place and importance in mathematics that can’t be ignored or avoided, and that it is a major step for the development of mathematics.

 

Infinities and not just one infinity

Well, there isn’t just one infinity, and not all of them are the same. In fact, there are infinities bigger than others or smaller than others, the most trivial way to illustrate it is to add a number to infinity and you get a bigger infinity: , but let’s see some examples of some infinities:

• The number of natural numbers is infinite: 0,1,2, 3, …,
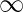
• The number of the Odd natural numbers is also infinite: 1,3,5,7,9, …,
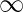
• The number of the Even natural numbers is also infinite: 0,2,4,6,8, …,
• In comparison both the Odd natural numbers and Even natural numbers are infinite and they are equally infinite since we can associate for every even number and odd number just by adding 1. But both the infinities are smaller than the number of natural numbers since this last infinity is the sum of the two infinities of odd and even numbers.
• The number of real numbers in the interval is infinite.
• Not just that but and while the interval is limited it contains an infinite number of real numbers.
• Also, this infinity (The number of real numbers in the interval ) is bigger than the number of the natural numbers .
• The set of real numbers is a bigger infinite set than the set of natural numbers .

In mathematics, infinity can be positive or negative, there is no number bigger than positive infinity, and no number smaller than negative infinity.

These differences between the various infinities are the reason why we have some indeterminate forms, for example, we can’t say that the division of infinity on infinity is 1 because they might be different infinities and one is bigger than the other, or the case of infinity minus infinity we can’t say that the result is 0, and that’s the reason why we resort to some other methods like L’Hôpital’s rule to avoid the indeterminate forms. Of course, we don’t face any problems in some operations on infinities like \infty)\infty\times\infty

   

\pi\pi\infty+1$ and the same thing for the other current guests” and that’s true, but we can still accommodate this infinity of guests, all we have to do is to move every person to the room with the number equal two times the current one meaning moving each guest for room n to room 2n (guest from room 1 to room 2, guest from room 2 to room 4, … etc.), and by doing this we assure that all the rooms with odd numbers are empty and which are infinite since the old residents of the hotel are now occupying only the rooms with even numbers, and therefore, we can accommodate the infinite guests in the infinite empty rooms with odd numbers.

How interesting is that!!! A full hotel can accommodate an infinity of new guests!!!

Well, there are many other cases treated by the Hilbert’s paradox of the Grand Hotel; check out these two videos for more interesting scenarios!!!

Zeno’s paradox (also called the dichotomy paradox)

Let’s suppose that a person wants to walk from one end of the room to the other end, to do so, he must first walk half the distance which will take a finite amount of time, once at the halfway point, he needs to walk half of the remaining distance which also takes a finite amount of time, after that he needs to walk half of the remaining distance which will also take an infinite amount of time and so forth. This means that he will take an infinite number of steps since any distance can be divided again and again with each step, and since he will need to take an infinite number of steps and each of these steps takes a finite amount of time then the time he will need to get to the other side of the room is the sum of the infinite number of finite amounts of time which should result in an infinite time, so if the total time needed to walk to the other side of the room is infinite then he will never reach the other side!!! This means that moving from any place to any other place should take an infinite amount of time and therefore motion is impossible!!!

Check out the solution for this paradox in this video

 

Conclusion

At the end of this article, we hope you enjoyed learning some things about the strange, amazing, and even confusing side of the concept of infinity. And remember “there is an infinity of things to learn about infinity”!!!

For more articles related to infinity, take a look at the post Limit of a function: Introduction, or Limits of a function: Operations and Properties, or the one about Limits of a function: Indeterminate Forms!!!!!

Also, if you want to learn more fun subjects, check the post about Functions and some of their properties, or the one about How to solve polynomial equations of first, second, and third degrees!!!!!

And don’t forget to join us on our Facebook page for any new articles and a lot more!!!!!