Probability: Terminology and Evaluating Probabilities

by ms-academy | Oct 24, 2021 | Math Learning

Table of Contents

After introducing the branch of probability theory in the previous article and presenting an explanation for what is a probability, the main types of probability, and some examples of the fields where it is used; in this article, we will learn about the terminology and how to determine the probability with some illustrating examples.

Introduction

After introducing the probability theory in the previous article: Probability: Introduction to Probability Theory, we continue our journey by diving more and learning the basics, in this article, we will continue the introduction and learn some terminology alongside the basic method of evaluating and calculating the probability of a given event.

Terminology

Experiment

We mean by experiment action of performing or conducting a test, an evaluation, or an investigation, and we get a result by the end of the experiment. an experiment may have one or many possible results, also, based on the number of possible results we can define two types of experiments: the deterministic experiments and the probabilistic ones.

Deterministic experiment:

We call a determinist experiment every experiment or a trial that we can predict with certainty the outcome experiment in advance, meaning throughout the experiment, there is no part ruled by chance or chaos and thus we can determine with certainty the outcome of the experiment even before it happens if we know its inputs or variables.

Examples of deterministic experiments:

The addition of two numbers $A$ and $B$ ; for instance, the addition of 8 and 21 is certainly 29, there is no maybe in there.
The multiplication of two numbers;
The experiment of decomposition of water, we know for sure that we will have in result oxygen and hydrogen.
Science experiments of established laws like conducting an experiment to test Newton’s laws Motion or gravity …etc.

Random experiment:

We call random experiment (or probabilistic experiment) every experiment or a trial where we can’t foretell the outcome of the experiment beforehand, in other terms, throughout the experiment, there is one or many parts of it ruled by chance and randomness and therefore we can’t predict with certainty the result of the experiments before the experiment is done.

Examples of random experiments:

Rolling a die, we know the possible results are the numbers from 1 to 6 but we can’t predict with certainty the result.
Tossing a coin, we know that there are two possible results Head or tails, but we can’t determine beforehand the outcome of a toss.
Selecting a numbered ball from an urn containing balls numbered from 1 to 100, of course without looking we can’t foretell the number of the ball we are going to take out.
Taking out a card from a well-shuffled deck of cards.
The winner of a car race.
The winner or winners of a lottery.
The score of a basketball game.

As we already know by now, probability theory is the study of the chance of occurrence of events, so we will be interested in studying the random experiment and trying to determine the different probabilities.

Trial

We call a trial the act of performing the experiment, therefore when we conduct a random experiment several times every one of them is called a trial.

Outcome

We call an outcome every possible result of the experiment.

For example: if the experiment is to roll a die then the possible outcomes are: 1, 2, 3, 4, 5, or 6.

Sample space

We call the sample space, the set containing all possible outcomes.

For example: for the experiment of rolling a die, the sample space is $S=\lbrace1,2,3,4,5,6\rbrace$ .

Event

An event $E$ is a set of outcomes of the experiment, or in other terms, an event is a subset of the sample space set, in other terms we can say that an event is a collection of outcomes that have a common property. if the result of the experiment is contained in $E$ we say that the event $E$ has occurred.

For example: let consider the experiment of rolling a die, and we are interested in the outcomes where the number we get is even; we have the sample space is $S=\lbrace 1,2,3,4,5,6\rbrace$ and if we denote the event of having an even number by $E$ we have $E=\lbrace 2,4,6\rbrace$ . in this example, the event $E$ is a collection of outcomes that have the same property which is being even.

Note that, if we consider two events $A$ and $B$ then their union $A\cup B$ and their intersection $A\cap B$ are also events.

Finding the probability of an event

Let’s suppose we have an experiment with its sample space, to determine the probability of a given event $E$ , we need to extract the important and essential information about the experiment, and then by using the probability axioms we proceed to evaluate the wanted probability.

Let’s take a look at an example:

If we take the experiment or roll a fair die, and we want to determine the probability of the event where we get an odd number, the information given is:

Fair die which leans that all the outcomes (numbers from 1-6) have the same chance of occurrence, therefore $P(1)=(2)=...=P(6)$ .
The event is getting an odd number, so we can note $E=\lbrace 1,3,5\rbrace$ .

Now since we have all the outcomes are equiprobable (meaning equally likely) we can to use the fundamental rule of evaluating probability:

$\text{The probability of occurrence of an event }P(E)= \dfrac{\text{The number of favorable outcomes}}{\text{The total number of outcomes}}$

In this case, we have the favorable outcomes are the outcomes that result in the occurrence of the event $E$ which means the favorable outcomes are: 1, 3, and 5 so we have three outcomes from the total number of outcomes which is 6.

Therefore, we have:

$\begin{align*} P(E) & =\dfrac{3}{6} \\ P(E) & =\dfrac{1}{2} \\ P(E) & =0.5 \\ P(E) & =50\% \end{align*}$

Note that the probability of the event $E$ where $E=\lbrace \rbrace=\emptyset$ is $P(\emptyset)=0$

Example 2: Let’s determine the probability of taking out an ace from a well-shuffled deck of cards.

So if we analyze the information we have:

The deck of cards contains 52 cards.
There are 4 aces.
The deck is well-shuffled therefore the chance of drawing any card is the same as any other card.

So by using the formula of probability we have:

The number of favorable outcomes is 4.
The number of the total outcomes is 52.

Therefore the probability of the drawing of an ace is 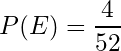$P(E)=\dfrac{4}{52}$ i.e. $P(E)=\dfrac{1}{13}$

Example 3: Let’s consider the event of getting at least one tail in three tosses of a fair coin.

The information we have are:

A fair coin thus the chance of having Head or Tail is the same.
The experiment consists of three tosses therefore the sample space is $S\lbrace HHH, HHT, HTH, HTT, THH, THT, TTH, TTT\rbrace$ .
The event we want to determine its probability is getting at least one Tail, meaning $E=\lbrace HHT, HTH, HTT, THH, THT, TTH, TTT\rbrace$ .

So by using the formula of probability we get:

The number of favorable outcomes is 7 (i.e. cardinal of $E$ ).
The number of the total outcomes is 8 (i.e. cardinal of $S$ ).

Therefore the probability of getting at least one tail after three tosses of a fair coin is 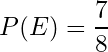$P(E)=\dfrac{7}{8}$ .

Conclusion

In this article, we learned the basic terminology of the probability theory; we also learned how to evaluate probabilities of given events by extracting the important and essential information and using the probability axioms to determine the probability of the event in question. keep in mind this is still an introduction to probability theory and we have much more to learn, and yes!!! there will be more articles on this subject!!!!!

In the meantime, you can take a look at some articles about limits and infinity like Limit of a function: Introduction, Limits of a function: Operations and Properties, Limits of a function: Indeterminate Forms, or the ones about Infinity: Facts, mysteries, paradoxes, and beyond or check the one about Probability: Introduction to Probability Theory!!!!!

Also, if you want to learn more fun subjects, check the post about Functions and some of their properties, or the one about How to solve polynomial equations of first, second, and third degrees!!!!!

And don’t forget to join us on our Facebook page for any new articles and a lot more!!!!!

← PREVIOUS NEXT →